Final Answer:
The trigonometric form of the complex number

Step-by-step explanation:
To express the complex number
 in trigonometric form, we utilize the polar form
in trigonometric form, we utilize the polar form
 where
where
 denotes the magnitude of the complex number, and
denotes the magnitude of the complex number, and
 represents the argument. First, calculating the magnitude
represents the argument. First, calculating the magnitude
 we take the absolute value of
we take the absolute value of
 , yielding
, yielding

This magnitude,
 , serves as the radial distance from the origin to the complex number in the complex plane. Next, determining the argument
, serves as the radial distance from the origin to the complex number in the complex plane. Next, determining the argument
 we consider the angle formed by the complex number. For
we consider the angle formed by the complex number. For
 the argument is
the argument is
 since the number lies along the negative imaginary axis.
since the number lies along the negative imaginary axis.
Substituting these values back into the polar form, we arrive at
 . In this representation, the magnitude
. In this representation, the magnitude
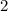 signifies the distance from the origin, and
signifies the distance from the origin, and
 denotes the angle with respect to the positive real axis. Thus, the trigonometric form succinctly conveys the complex number's geometric properties in the polar coordinate system, providing both magnitude and direction information. In summary,
denotes the angle with respect to the positive real axis. Thus, the trigonometric form succinctly conveys the complex number's geometric properties in the polar coordinate system, providing both magnitude and direction information. In summary,
 serves as a concise and precise representation of the complex number
serves as a concise and precise representation of the complex number
 in trigonometric form.
in trigonometric form.