The total work required to pump all the water to a height of 5 meters above the tank is approximately
 joules (since work is measured in joules in the SI system).
joules (since work is measured in joules in the SI system).
To calculate the work required to pump all the water to a height of 5 meters above the tank, we need to use the concept of work from physics, where work is the product of force and distance. The force, in this case, is the weight of the water, which is the product of the volume of the water, the density of water, and the acceleration due to gravity.
The work done to lift a thin slice of water at a certain height to the top of the tank is given by the formula:
![\[ \text{Work} = \text{Force} * \text{Distance} \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/y33396ujcjych7ir3mqanv56igtewc9m6x.png)
![\[ \text{Work} = (\text{Volume of water slice}) * (\text{Density of water}) * (g) * (\text{Distance to top of tank}) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/9mcgw7ssoj44go6tyl0e19jedv9n9th0h1.png)
Since the tank is hemispherical, we'll integrate over the height of the hemisphere to find the total work done.
First, let's consider a thin slice of water at height
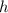 with a thickness
with a thickness
 . The volume of this slice,
. The volume of this slice,
 , is the area of the circle at height
, is the area of the circle at height
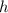 times
times
 , and the area
, and the area
 is
is
 , where
, where
 is the radius of the circle at height
is the radius of the circle at height
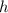 . Using the Pythagorean theorem, we know that
. Using the Pythagorean theorem, we know that
 , where \( R \) is the radius of the hemisphere (8 m).
, where \( R \) is the radius of the hemisphere (8 m).
1. Calculate the radius of the circular slice at height \( h \):
![\[ r(h) = √(R^2 - (R - h)^2) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/z17tey3uz6ecjmo82uf8afwa4pqow7a5ac.png)
2. Calculate the volume of the thin slice:
![\[ dV = A \cdot dh = \pi r(h)^2 \cdot dh \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/kq1ms7oyvwr5m5spcm9e31s2lygqcyvkad.png)
3. Calculate the force exerted by the water slice, which is its weight:
![\[ dF = dV \cdot \text{density} \cdot g \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/69otl3g3yv0di3qh10bvkbt8hadpv5s5bl.png)
The density of water is
 and
and
 (acceleration due to gravity) is
(acceleration due to gravity) is
 .
.
4. The distance that each slice must be lifted is \( 5 \text{ m} - h \), since the tank itself is 8 meters high and we need to lift the water 5 meters above the tank, the total lifting height is \( 13 - h \) meters.
5. Calculate the work for the thin slice:
![\[ dW = dF \cdot (5 \text{ m} - h) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/h2ouoo51ax01pbs7g5siwctb88rlxb6gm8.png)
6. Integrate this over the height of the hemisphere, from \( 0 \) to \( R \):
![\[ W = \int_0^R dW \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/8z8bomxwcnktyuvkqra9qnynu2s7vv3dkg.png)
![\[ W = \int_0^R \pi (R^2 - (R - h)^2) \cdot 1000 \cdot 9.8 \cdot (5 - h) \, dh \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/un2gvzv6f3l6hxcnz1lxyo1keup59pzek2.png)
Let's perform this integration to find the total work done.
7. Integrate and simplify:
![\[ W = 9800\pi \int_0^8 (64 - (8 - h)^2)(5 - h) \, dh \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/dmluis8kslsqn3ss8cm4fzdc6k0lqykuqx.png)
The total work required to pump all the water to a height of 5 meters above the tank is approximately
 joules (since work is measured in joules in the SI system).
joules (since work is measured in joules in the SI system).