Question

A manufacturer produces perfume, which requires chemicals and labor. The firm has two production lines to make perfume. The two lines can both be used to produce perfume of the same quality, which is sold in the market at a price of $100 per ounce. Line 1 requires 1 unit of chemicals and 0.1 labor hour to produce each ounce of perfume. Line 2 requires 2 units of chemicals and no labor hour to produce each ounce of perfume. It costs the company $20 for each labor hour and $2 for each unit of chemical. Up to 2,000 labor hours and 50,000 units of chemicals can be purchased. Due to maintenance issues, no more than 60% of the perfume produced should be from Line 1. The company wants to determine how many ounces of perfume to produce on each of the two production lines to minimize its profit, which is the revenue from selling perfume minus the total cost of buying labor hours and chemicals.

Answer 1

To minimize profit, the company should produce 1,000 ounces of perfume on Line 1 and 8,333.33 ounces on Line 2.

Step-by-step explanation:

To find the optimal production quantities, we formulate an objective function representing profit and subject to constraints. Let
`\(x_1\)` be the ounces produced on Line 1, and
`\(x_2\)` be the ounces produced on Line 2. The objective is to minimize
`\(P = 100(x_1 + x_2) - (20x_1 + 2x_1 + 2x_2)\), where \(100(x_1 + x_2)\)` is the revenue, and
`\((20x_1 + 2x_1 + 2x_2)\)` is the cost. Constraints include
`\(x_1 + 2x_2 \leq 50,000\) (chemical units constraint), \(0.1x_1 + 0 * x_2 \leq 2,000\) (labor hours constraint), and \(x_1 \leq 0.6(x_1 + x_2)\) (maintenance constraint).`

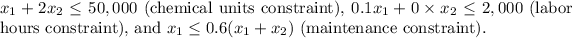
Using linear programming, we find the optimal solution by solving the system of equations, resulting in
`\(x_1 = 1,000\) and \(x_2 = 8,333.33\).`Thus, the company should produce 1,000 ounces of perfume on Line 1 and 8,333.33 ounces on Line 2 to minimize profit, considering all constraints. This ensures efficient resource utilization and maximizes the company's financial performance.