Final Answer:
It will take approximately 8 years and 2 months to save $2329.00 by depositing $69.00 at the end of every three months into an account earning 5% interest compounded quarterly.
Step-by-step explanation:
To calculate the time needed to save $2329.00 by making deposits of $69.00 at the end of every three months at a 5% interest rate compounded quarterly, the formula for compound interest needs to be applied. The formula used here is
 , where A represents the amount of money accumulated after n years, P is the principal amount (the initial deposit), r is the annual interest rate (in decimal), n is the number of times that interest is compounded per year, and t is the time in years.
, where A represents the amount of money accumulated after n years, P is the principal amount (the initial deposit), r is the annual interest rate (in decimal), n is the number of times that interest is compounded per year, and t is the time in years.
Given:
P = $69.00 (deposit made at the end of every three months)
r = 5% or 0.05 (annual interest rate)
n = 4 (compounded quarterly)
A = $2329.00 (target amount)
Using the formula and solving for t:
2329 = 69(1 + 0.05/4)
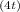
By rearranging the equation to solve for t:
(1 + 0.0125)
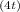 = 2329 / 69
= 2329 / 69
(1.0125)
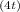 = 33.7246
= 33.7246
4t ≈ log(33.7246) / log(1.0125)
t ≈ log(33.7246) / (4 * log(1.0125))
t ≈ 8.17 years
Thus, it will take approximately 8 years and 2 months to save $2329.00 with these deposits and interest rates.