Question

You bu a new mountain bike for $200. The value of the bike decreases by 25% each year. a. Write a model giving the mountain bike's value of the bike after 3 years b. grpah the model Estimate when the balue of the bike will be $100

Answer 1

a. The mountain bike's value after 3 years is approximately $112.50.

b. The value of the bike will be $100 after approximately 4.96 years.

Step-by-step explanation:

a. To find the value of the mountain bike after 3 years with a 25% decrease each year, we use the formula for exponential decay:
`\( V_t = P * (1 - r)^t \)`, where
`\( V_t \)` is the value after ( t ) years, ( P ) is the initial price, ( r ) is the rate of decrease (expressed as a decimal), and ( t ) is the number of years. Plugging in the values, we get
`\( V_3 = 200 * (1 - 0.25)^3 \)`, which simplifies to
`\( V_3 = 200 * (0.75)^3 \), resulting in \( V_3 \approx 112.50 \).`

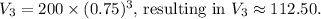
b. To estimate when the value of the bike will be $100, we set
`\( V_t = 100 \)` in the decay formula and solve for ( t ):
`\( 100 = 200 * (0.75)^t \).` Dividing both sides by 200, we get
`\( 0.5 = (0.75)^t \)`. Taking the natural logarithm of both sides, we have
`\( \ln(0.5) = t * \ln(0.75) \)`. Solving for ( t ), we find
`\( t \approx 4.96 \)` years.

In summary, after 3 years, the mountain bike's value will be approximately $112.50. To reach a value of $100, it will take approximately 4.96 years. The exponential decay formula allows us to model the decreasing value of the bike over time, providing a clear understanding of its depreciation.