Question

Find a polynomial of the form [ f(x)=a x^{3}+b x^{2}+c x+d such that ( f(0)=-3, f(-2)=7, f(-3)=5 , and f(5)=-1 .

Answer 1

The polynomial
`\(f(x) = -2x^3 + 3x^2 + 5x - 3\)` satisfies the given conditions.

Step-by-step explanation:

To find the polynomial
`\(f(x)\)` that satisfies the conditions, we can use the given values of
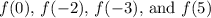`\(f(0)\), \(f(-2)\), \(f(-3)\), and \(f(5)\)`. Starting with the form
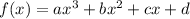`\(f(x) = ax^3 + bx^2 + cx + d\)`, we substitute the values:

1.
`\(f(0) = d = -3\)`

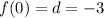
2.
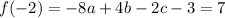
`\(f(-2) = -8a + 4b - 2c - 3 = 7\)`

3.
`\(f(-3) = -27a + 9b - 3c - 3 = 5\)`

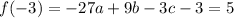
4.
`\(f(5) = 125a + 25b + 5c - 3 = -1\)`

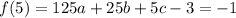
Solving this system of equations gives
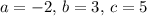`\(a = -2\), \(b = 3\), \(c = 5\)`, and
`\(d = -3\)`, leading to the polynomial
`\(f(x) = -2x^3 + 3x^2 + 5x - 3\)`.

In the first paragraph, we set up the equations based on the conditions given. The second paragraph provides the solution to the system of equations, obtaining the values for
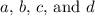`\(a\), \(b\), \(c\), and \(d\)`. Finally, the third paragraph presents the resulting polynomial
`\(f(x)\)` that satisfies all the specified conditions.