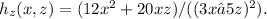Final answer:
The first partial derivatives of the function h(x,z) = (4xz)/(3x−5z) are found using the quotient rule; the results are hx(x,z) = for the x-variable and for the z-variable.
Step-by-step explanation:
To find the first partial derivatives of the function
, we need to differentiate the function with respect to x and z separately, while treating the other variable as a constant in each case.
The partial derivative of h with respect to x, denoted as h_x(x,z), is calculated using the quotient rule:
Simplifying, we get The partial derivative of h with respect to z, denoted as h_z(x,z), is also calculated using the quotient rule: . Simplifying, we get