Final Answer:
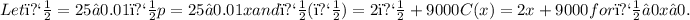
Step-by-step explanation:
The given expressions represent functions involving a variable x. The function p = 25 - 0.01x describes a linear relationship where the value of p is determined by the value of x. Specifically, p equals 25 minus 0.01 times x. This suggests that as x increases, p decreases at a rate of 0.01 times x.
On the other hand, C(x) = 2x + 9000 is a linear cost function. It represents the cost C(x) associated with a certain quantity x, where the cost is determined by multiplying x by 2 and adding a constant term of 9000. The term 2x accounts for a variable cost that increases proportionally with x, and the constant 9000 represents a fixed cost. This function is valid for x ≥ 0, meaning it applies for non-negative values of x.
Understanding these functions is essential for analyzing relationships in various scenarios, such as business or economics, where the variables p and C could represent prices and costs, respectively. The linear nature of these functions allows for straightforward interpretation and mathematical analysis to derive insights into the underlying relationships between the variables.