Question

Find the absolute maximum value on (0, [infinity]) for f(x)=11-12 x-12/x

Answer 1

The absolute maximum value of the function
`\(f(x) = 11 - (12x)/(x)\)` on the interval
`\((0, \infty)\) is \(11\).`

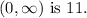
Step-by-step explanation:

In order to find the absolute maximum value of f(x) on the given interval, we need to analyze the behavior of the function. As (x) approaches infinity, the term
`\(-(12)/(x)\)` tends towards zero. Therefore, the dominant term in the function becomes \(11\), and the function approaches (11) as (x) goes to infinity.

To express this mathematically, as (x) approaches infinity,
`\(f(x) = 11 - (12)/(x) \rightarrow 11\)`. This indicates that the function f(x) has an asymptote at (y = 11\), and as (x) becomes arbitrarily large, f(x) gets arbitrarily close to (11). Thus, the absolute maximum value of (f(x) on the interval
`\((0, \infty)\) is \(11\).`

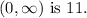
It's important to note that for any value of (x) within the given interval, the function f(x) will always be less than or equal to (11). This is because the second term,
`\(-(12)/(x)\)`, is always subtracted from (11). Therefore, (11) is not just a maximum but the absolute maximum value, as no other value within the specified domain surpasses it.