Question

Choose the correct formula for the function h. h(x)=-3^(x+2)-1 h(x)=-3^(x-2)-1³ˣ⁻²

Answer 1

The correct formula for the function h is h(x) =
`-3^(x+2) - 1.`

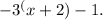
Step-by-step explanation

In the given function h(x) =
`-3^(x+2) - 1,` the exponent is (x+2). This means that the base, -3, is raised to the power of (x+2). The negative sign outside the exponent applies to the entire term, indicating that the result of
`-3^(x+2)` is negated. The final term, -1, is then subtracted from this result.

To break it down further, let's consider the exponent (x+2). This implies that whatever value x takes, it is added to 2 before being used as the exponent. For instance, if x is 1, the exponent becomes (1+2) = 3. If x is 0, the exponent is (0+2) = 2. This addition of 2 to x inside the exponent distinguishes it from other possible formulations.

Therefore, the correct representation of the given function is
`h(x) = -3^(x+2) - 1,` where the negative sign applies to the entire term
`-3^(x+2)`, and then 1 is subtracted from the result. This formula accurately reflects the mathematical relationship described in the function h.