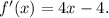Final Answer:
By using the limit definition of the derivative can be find as
![\[ (d)/(dx) (2x^2 - 4x) = 4x - 4 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/jl0scj4g1wk6at2y9r3gpmytd0ngw107vg.png)
Step-by-step explanation:
The limit definition of the derivative is given by the formula:
![\[ f'(x) = \lim_{{h \to 0}} (f(x + h) - f(x))/(h) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/u6hl930osxfdo0211qu2kr99q3eubokoao.png)
For the given function
 , let's apply the limit definition to find its derivative f'(x):
, let's apply the limit definition to find its derivative f'(x):
![\[ f'(x) = \lim_{{h \to 0}} ((2(x + h)^2 - 4(x + h)) - (2x^2 - 4x))/(h) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/a2s2g3q37sdqgzh5j17vpxl1o8quvgd7qh.png)
Now, let's expand and simplify the numerator:
![\[ f'(x) = \lim_{{h \to 0}} (2(x^2 + 2xh + h^2) - 4(x + h) - 2x^2 + 4x)/(h) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/zvrw70g5tb780ba8yf7qeom7grobdcpqz3.png)
Combine like terms:
![\[ f'(x) = \lim_{{h \to 0}} (2x^2 + 4xh + 2h^2 - 4x - 4h - 2x^2 + 4x)/(h) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/46zxhe2pvui6zhwtgp51uzaumov9hi5q4t.png)
Cancel out common terms:
![\[ f'(x) = \lim_{{h \to 0}} (4xh + 2h^2 - 4h)/(h) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/2m5crocglvhkdc1nzok4qt9mgv2zcym6jn.png)
Now, factor out h from the numerator:
![\[ f'(x) = \lim_{{h \to 0}} (h(4x + 2h - 4))/(h) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/gsqfd0ordoc9ssl293cs13hf1x5l6oxush.png)
Cancel h from the numerator and denominator:
![\[ f'(x) = \lim_{{h \to 0}} (4x + 2h - 4) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/sjoatcyjcm9gvzhgqafn58tkjhl83rk9vy.png)
Now, substitute h = 0 :
![\[ f'(x) = 4x - 4 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/ycv96fjg6d366tm9wudnioaa9r4iivx2vz.png)
So, the derivative of
 is
is