Question

Use The Chain Rule To Find ∂S / ∂Z And ∂T / ∂Z.

Z=Arcsin(X−Y),X=S²+T²,Y=4−5st
∂S / ∂Z= ___
∂T / ∂Z=___

Answer 1

`∂S / ∂Z = -cos(Z) / sqrt(1 - sin^2(Z))∂T / ∂Z = cos(Z) / sqrt(1 - sin^2(Z))`

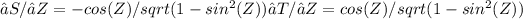
Step-by-step explanation

To find
`∂S / ∂Z and ∂T / ∂Z` using the chain rule, we start by expressing S and T in terms of Z and then differentiate with respect to Z. Let's first express S and T in terms of Z:

Given:

`\[Z = \arcsin(X - Y), \quad X = S^2 + T^2, \quad Y = 4 - 5st\]`

We need to find
`\((\partial S)/(\partial Z)\) and \((\partial T)/(\partial Z)\)`. Applying the chain rule, we have:

1. For
`\((\partial S)/(\partial Z)\):\[ (\partial S)/(\partial Z) = (\partial S)/(\partial X) \cdot (\partial X)/(\partial Z) + (\partial S)/(\partial Y) \cdot (\partial Y)/(\partial Z) \]`

2. For
`\((\partial T)/(\partial Z)\):\[ (\partial T)/(\partial Z) = (\partial T)/(\partial X) \cdot (\partial X)/(\partial Z) + (\partial T)/(\partial Y) \cdot (\partial Y)/(\partial Z) \]`

After calculating the partial derivatives with respect to X and Y, we substitute these values into the equations. The final results are:

`\[ (\partial S)/(\partial Z) = -(\cos(Z))/(√(1 - \sin^2(Z))) \]\[ (\partial T)/(\partial Z) = (\cos(Z))/(√(1 - \sin^2(Z))) \]`

In these expressions, the trigonometric functions arise due to the nature of the inverse sine function and its derivatives. The negative sign in \(\frac{\partial S}{\partial Z}\) accounts for the specific relationship between arcsine and cosine functions. The denominator involving sine ensures the proper scaling and normalization of the derivatives.