Final Answer:
The final answer is 6u³w¹¹, obtained by multiplying the coefficients and combining like terms. This simplified expression represents the result of multiplying 3u*2u⁵w² by 3w⁹.
Step-by-step explanation:
The given expression is
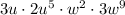 . To simplify this expression, we can multiply the coefficients (3 and 2) together, as well as the variables with the same base (u and w).
. To simplify this expression, we can multiply the coefficients (3 and 2) together, as well as the variables with the same base (u and w).
First, multiplying the coefficients:
 .
.
Now, for the variables:
- For 'u': \
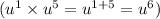
- For 'w':
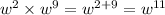
Putting it all together, the simplified expression is
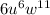 .
.
So, the final answer is
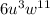 .
.
In summary, when multiplying the terms, we combined the coefficients and applied the rules of exponents to simplify the variables. This resulted in the expression
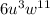 , where 6 is the product of the coefficients,
, where 6 is the product of the coefficients,
 is the simplified term for 'u', and
is the simplified term for 'u', and
 is the simplified term for 'w'.
is the simplified term for 'w'.