Final Answer:
The relative maximum value of the function
 subject to the constraint
subject to the constraint
 occurs at the point
occurs at the point
 with a maximum value of
with a maximum value of
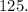
Step-by-step explanation:
Certainly! To find the relative maximum value of the function
 subject to the constraint
subject to the constraint
 we can use the method of Lagrange multipliers.
we can use the method of Lagrange multipliers.
1. Formulating the Lagrangian:
The Lagrangian function is given by:
![\[L(x, y, \lambda) = x^2 y + \lambda(10 - x - y)\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/4w894ae7lmw9eywjczonm4zi7rpdxpv52n.png)
2. Taking Partial Derivatives:
Calculate the partial derivatives of
 and set them equal to zero:
and set them equal to zero:
![\[(\partial L)/(\partial x) = 2xy - \lambda = 0\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/7mspossa9it1oh22r5fyd1na7xvr7zupy6.png)
![\[(\partial L)/(\partial y) = x^2 - \lambda = 0\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/hkyfy1honrqi1t2poaoji5esm0gli9holb.png)
![\[(\partial L)/(\partial \lambda) = 10 - x - y = 0\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/wuzegg0e1couzgv810zl3j8581hhx9etsk.png)
3. Solving the System of Equations:
Solve the system of equations to find the critical points. From the first two equations, we get
 Substituting into the third equation
Substituting into the third equation
 we find
we find
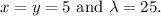
4. Substitute into the Objective Function:
Substitute these values back into the objective function
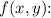
![\[f(5, 5) = 5^2 * 5 = 125\]](https://img.qammunity.org/2024/formulas/mathematics/high-school/i2uq7qeh37o0kkinh3qmq6354emqugsa9e.png)
Therefore, the relative maximum value of the function
 subject to the constraint
subject to the constraint
 occurs at the point
occurs at the point
 with a maximum value of
with a maximum value of
 . This point satisfies both the original function and the constraint, and the Lagrange multipliers method helps identify critical points for optimization problems with constraints.
. This point satisfies both the original function and the constraint, and the Lagrange multipliers method helps identify critical points for optimization problems with constraints.