Question

Mar 07, 9:56:51 AM Which of the following values are solutions to the inequality 9<=-7+4x?

Answer 1

The values that are solutions to the inequality
`\(9 \leq -7 + 4x\)` are
`\(x \geq 4\).`

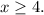
Step-by-step explanation:

The inequality
`\(9 \leq -7 + 4x\)` can be solved step by step to find the values of x that satisfy the inequality. First, add 7 to both sides to isolate the term with 4x:

`\[ 9 + 7 \leq -7 + 7 + 4x \]`

This simplifies to
`\(16 \leq 4x\)`. Next, divide both sides by 4 to solve for x:

`\[ (16)/(4) \leq (4x)/(4) \]`

Simplifying further, we get
`\(4 \leq x\)`. Therefore, the final answer is
`\(x \geq 4\).`

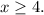
In practical terms, this means any value of x that is greater than or equal to 4 will satisfy the original inequality. For instance, if x is 4 or any value greater than 4, the inequality will hold true. However, if x is less than 4, the inequality will not be satisfied.

In conclusion, the solution to the inequality
`\(9 \leq -7 + 4x\)` is
`\(x \geq 4\),` indicating a range of values for x that make the inequality true. This solution is derived through algebraic manipulations, ensuring a systematic and accurate determination of the variable's possible values.