Answer:
x₂ = 4.455 m
Step-by-step explanation:
The average speed is defined as the displacement traveled between the time interval
v_average =
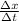
in this case we have a first stop walking west at speed v = 3.10 m / s a distance of x = 5.96 km = 5.96 10³ m
Let's find the time it takes on this tour
v = x / t
t = x / v
t₁ = 5.96 10³ / 3.10
t₁ = 1.9226 10³ s
in a second to walk east at a speed of v₂ = 0.744 m / s
v₂ = x₂ / t₂
t₂ = x₂ / v₂
for full movement
let's assume that the eastward movement is positive
v =
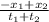
v =
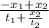
the only unknown term is the distance to the east. We replace and resolve
1.19 =
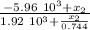
1.19 (1.92 10³ +
 ) = x₂ 1.92 10³ - 5.96 10³
) = x₂ 1.92 10³ - 5.96 10³
2.2848 10³ + 1.599 x₂ = 1.92 10³ x₂ - 5.96 10³
x₂ (1.92 10³ - 1.599) = 2.2848 10³ + 5.96 10³
x₂ = 8.5448 10³ / 1.918 10³
x₂ = 4.455 m