The opposites of square roots are
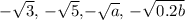 .
.
To express the opposite of a square root, you can use the negation outside the radical symbol. Let's rewrite each expression:
1.
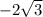 : The opposite is
: The opposite is
 .
.
2.
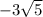 : The opposite is
: The opposite is
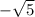 .
.
3.
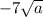 : The opposite is
: The opposite is
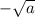 .
.
4.
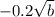 : The opposite is
: The opposite is
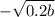 .
.
In each case, the negation is applied directly to the square root term. It's important to note that the negation changes the sign of the entire expression within the radical, while the radical itself remains unchanged.
This transformation reflects the concept of taking the opposite or negation of a quantity represented by the square root.