Question

A national health report indicates that 75% of children ages 2 to 17 in the U.S. have seen a dentist in the past year. A dentist in Boston wanted to see if the city had the same percentage as the nation. In a sample of 125 children living in Boston, 95 reported seeing a dentist in the past year. Perform a hypothesis test to determine if the % in Boston is different than the national %, use alpha=0.05.

Answer 1

Perform a two-sample z-test for proportions to compare the percentage of children in Boston seeing a dentist to the national percentage of 75%.

The null hypothesis (H0) is that the percentage of children in Boston (p Boston​ ) is equal to the national percentage ( p national​ ), while the alternative hypothesis (H1) is that p Boston is different from p national

​The sample data from Boston is as follows:

Sample size in Boston ( n Boston ): 125

Number of children in Boston who have seen a dentist (x Boston ): 95

Sample proportion in Boston ( Boston / n Boston = 95/125

​The national percentage (p national ) is given as 75%.

First, check the conditions for the z-test:

Random sampling: Assume that the sample is randomly selected.

Independence: The sample should be less than 10% of the population.

Success-failure condition: Ensure that both n Boston ⋅p Boston and ​(1− p^Boston ) are greater than 5.

Next, calculate the test statistic (z) using the formula:

z =
`(p Boston-p National​)/(√(pNational(1-pNational)/nBoston) )`

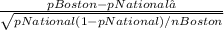
Then, find the p-value associated with z and compare it to the significance level (α=0.05).

If the p-value is less than or equal to α, reject the null hypothesis; otherwise, fail to reject it.

This test will help determine if the percentage of children in Boston seeing a dentist is significantly different from the national percentage of 75%.