Question

Let the zero point of potential energy be where the mass on the pendulum is at its lowest point. Calculate the total energy. Answer in units of J.

Answer 1

The total energy of the pendulum at the lowest point, where the potential energy is considered zero, is the sum of its kinetic energy and potential energy. It can be represented as the total energy (E) = kinetic energy (KE) + potential energy (PE).

Step-by-step explanation:

At the lowest point of the pendulum, where the potential energy is set to zero, all the energy is in the form of kinetic energy. The total energy (E) is the sum of the kinetic energy (KE) and potential energy (PE). The formula for the total energy of a pendulum is E = KE + PE. Since the potential energy is considered zero at the lowest point, the total energy is solely the kinetic energy at this point.

The formula for kinetic energy (KE) is KE = 1/2 × mass ×
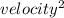`velocity^2`. At the lowest point, the velocity is at its maximum, and the potential energy is zero. Hence, the total energy (E) is equal to the kinetic energy (KE) alone. If the mass and velocity of the pendulum are provided, the total energy can be calculated using the kinetic energy formula.

In summary, when the potential energy is assumed to be zero at the lowest point of the pendulum's swing, the total energy is entirely in the form of kinetic energy. Therefore, the total energy can be determined using the kinetic energy formula, considering the maximum velocity of the mass at the lowest point.