Question

What is the probability that you will get all six cell phone companies ranked in the correct order?

Answer 1

The probability that you will get all six cell phone companies ranked in the correct order is
`\( (1)/(720) \).`

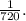
The probability of correctly ordering all six companies is a permutation problem.

There are 6! (read as "6 factorial") ways to arrange the companies, but only one of those arrangements is correct.

Therefore, the probability (P) is given by:

`\[ P = \frac{\text{Number of favorable outcomes}}{\text{Total number of outcomes}} = (1)/(6!) \]`

Simplifying, we get
`\( (1)/(720) \).`.

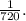Therefore, the correct answer is (a)
`\( (1)/(720) \).`

The probable question may be:

The teacher assigns a project where students are required to rank six different cell phone companies in their order of preference. The task is to calculate the probability of a student correctly ranking all six cell phone companies in the correct order.

a) \( \frac{1}{720} \)

b) \( \frac{1}{120} \)

c) \( \frac{1}{24} \)

d) \( \frac{1}{6} \)