Question

Solve: The formula P = 0.61x2 - 0.048x + 2 models the approximate population P, in thousands, for a species of fish in a

local pond, x years after 1997. During what year will the population reach 40,656 fish?

Answer 1

The population will reach 40,656 fish approximately 503.92 years after 1997, which corresponds to the year 2036.

To find the year in which the population will reach 40,656 fish, we need to solve the equation
`P = 0.61x^2 - 0.048x + 2`.

We set P equal to 40.656 and solve for x.

`0.61x^2 - 0.048x + 2 = 40.656`

`0.61x^2 - 0.048x - 38.656 = 0`

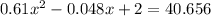
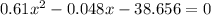
Using the quadratic formula, x = (-(-0.048) ± √((-0.048)^2 - 4(0.61)(-38.656))) / (2(0.61))

x ≈ 503.92 or x ≈ -1.06

Since x represents the number of years after 1997, the population will reach 40,656 fish approximately 503.92 years after 1997.

This corresponds to the year 2036.