Question

Seventy-two percent of the light aircraft that disappear while in flight in a certain country are subsequently discovered. Of the aircraft that are discovered, 66% have an emergency locator, whereas 80% of the aircraft not discovered do not have such a locator. Suppose a light aircraft has disappeared. (Round your answers to three decimal places.)

a) If it has an emergency locator, what is the probability that it will not be discovered?
b) If it does not have an emergency locator, what is the probability that it will be discovered?
c) If we consider 10 light aircraft that disappeared in flight with an emergency recorder, what is the probability that 7 of them are discovered?

Answer 1

a) 0.105 = 10.5% probability that it will not be discovered if it has an emergency locator.

b) 0.522 = 52.2% probability that it will be discovered if it does not have an emergency locator.

c) 0.064 = 6.4% probability that 7 of them are discovered.

Explanation:

For itens a and b, we use conditional probability.

For item c, we use the binomial distribution along with the conditional probability.

Conditional Probability

We use the conditional probability formula to solve this question. It is

`P(B|A) = (P(A \cap B))/(P(A))`

In which

P(B|A) is the probability of event B happening, given that A happened.

`P(A \cap B)` is the probability of both A and B happening.

P(A) is the probability of A happening.

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

In which
`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

And p is the probability of X happening.

a) If it has an emergency locator, what is the probability that it will not be discovered?

Event A: Has an emergency locator

Event B: Not located.

Probability of having an emergency locator:

66% of 72%(Are discovered).

20% of 100 - 72 = 28%(not discovered). So

`P(A) = 0.66*0.72 + 0.2*0.28 = 0.5312`

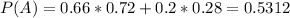
Probability of having an emergency locator and not being discovered:

20% of 28%. So

`P(A cap B) = 0.2*0.28 = 0.056`

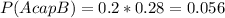
Probability:

`P(B|A) = (P(A \cap B))/(P(A)) = (0.056)/(0.5312) = 0.105`

0.105 = 10.5% probability that it will not be discovered if it has an emergency locator.

b) If it does not have an emergency locator, what is the probability that it will be discovered?

Probability of not having an emergency locator:

0.5312 of having. So

`P(A) = 1 - 0.5312 = 0.4688`

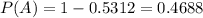
Probability of not having an emergency locator and being discovered:

34% of 72%. So

`P(A \cap B) = 0.34*0.72 = 0.2448`

Probability:

`P(B|A) = (P(A \cap B))/(P(A)) = (0.2448)/(0.4688) = 0.522`

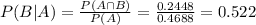
0.522 = 52.2% probability that it will be discovered if it does not have an emergency locator.

c) If we consider 10 light aircraft that disappeared in flight with an emergency recorder, what is the probability that 7 of them are discovered?

p is the probability of being discovered with the emergency recorder:

0.5312 probability of having the emergency recorder.

Probability of having the emergency recorder and being located:

66% of 72%. So

`P(A \cap B) = 0.66*0.72 = 0.4752`

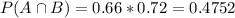
Probability of being discovered, given that it has the emergency recorder:

`p = P(B|A) = (P(A \cap B))/(P(A)) = (0.4752)/(0.5312) = 0.8946`

This question asks for P(X = 7) when
`n = 10`. So

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

`P(X = 7) = C_(10,7).(0.8946)^(7).(0.1054)^(3) = 0.064`

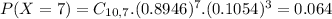
0.064 = 6.4% probability that 7 of them are discovered.