Question

I attended our industry's showcase conference last month and learned that Chester is updating their pricing strategy for their product Cent. Early intel suggests that they'll drop the price $1.80. They'll likely continue to keep both material and labor costs consistent at $15.23 and $11.43 per unit respectively, and I want us to better understand what their break-even point is. How many units (000) of Cent must they sell to break even? You can expect their period costs to stay consistent with the Income Statement available in your Drive. Thanks in advance! Sincerely, Sanjay Sanjay Singh Board Member, Operations | Andrews Corporation 453 units 538 units 589 units O 226 units ​

Answer 1

To find Chester's break-even point for Cent after a $1.80 price drop: Break-Even Point = Fixed Costs / (Current Selling Price - $1.80 - Variable Costs).

To determine the break-even point for Chester's product Cent after the expected price drop of $1.80, we use the break-even formula:
`\( \text{Break-Even Point (in units)} = \frac{\text{Fixed Costs}}{\text{Selling Price per Unit} - \text{Variable Costs per Unit}} \).`

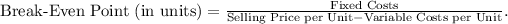
Firstly, find the selling price per unit after the expected price drop by subtracting $1.80 from the current selling price. The variable costs per unit include both material and labor costs, which sum up to $15.23 + $11.43. Fixed costs can be extracted from the Income Statement available in your Drive. Plug these values into the formula to calculate the break-even point in units.

Understanding the break-even point is crucial as it represents the quantity of units that need to be sold to cover all costs and reach a zero-profit situation. This information aids in decision-making, pricing strategies, and financial planning. Without the specific values for the current selling price and fixed costs, an exact break-even point can't be provided, but with the actual figures, the formula will yield the precise number of units Chester must sell to break even.