Question

Two professors are applying for grants. Professor Jane has a probability of 0.61 of being funded. Professor Joe has probability 0.29 of being funded. Since the grants are submitted to two different federal agencies, assume the outcomes for each grant are independent. Give your answer to four decimal places.

Required:
a. What is the probability that both professors get their grantsfunded? Give your answer to four decimal places.
b. What is the probability that at least one of the professors will befunded? Give your answer to four decimal places.
c. What is the probability that Professor Jane is funded but ProfessorJoe is not? Give your answer to four decimal places.
d. Given at least one of the professors is funded, what is theprobability that Professor Jane is funded but Professor Joe is not?

Answer 1

a) 0.1769 = 17.69% probability that both professors get their grants funded.

b) 0.7231 = 72.31% probability that at least one of the professors will be funded.

c) 0.4331 = 43.31% probability that Professor Jane is funded but Professor Joe is not.

d) 0.5989 = 59.89% probability that Professor Jane is funded but Professor Joe is not

Explanation:

a. What is the probability that both professors get their grants funded?

Jane has a 0.61 probability of being funded.

Joe has a 0.29 probability of being funded.

So

`p = 0.61*0.29 = 0.1769`

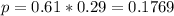
0.1769 = 17.69% probability that both professors get their grants funded.

b. What is the probability that at least one of the professors will be funded?

None:

Jane has a 1 - 0.61 = 0.39 probability of not being funded.

Joe has a 1 - 0.29 = 0.71 probability of not being funded.

0.39*0.71 = 0.2769

At least one:

1 - 0.2769 = 0.7231

0.7231 = 72.31% probability that at least one of the professors will be funded.

c. What is the probability that Professor Jane is funded but Professor Joe is not?

Jane has a 0.61 probability of being funded.

Joe has a 1 - 0.29 = 0.71 probability of not being funded.

0.61*0.71 = 0.4331

0.4331 = 43.31% probability that Professor Jane is funded but Professor Joe is not.

d. Given at least one of the professors is funded, what is the probability that Professor Jane is funded but Professor Joe is not?

0.4331/0.7231 = 0.5989

0.5989 = 59.89% probability that Professor Jane is funded but Professor Joe is not