Question

Drag each tile to the correct box

Consider this function.
1(x) = 5*
How is function / transformed to create function g? Match each transformation of function / with its description.
g(x)
53
g(z): 5t=
g(x)
vertical stretch of a factor of 3
horizontal stretch of a factor of 3
horizontal compression of a factor of
vertical compression of a factor of
(5)=
g(z)= 3(5)
>
1000

Answer 1

The function
`\( f(x) = 5^x \)` undergoes transformations to create function g(x). A vertical stretch by a factor of 3 corresponds to
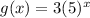`\( g(x) = 3(5)^x \)`, and a vertical compression by 1/3 corresponds to
`\( g(x) = (1)/(3)(5)^x \)`.

To identify how the function
`\( f(x) = 5^x \)` is transformed to create function g(x), let's match each transformation with its description:

A. **Vertical stretch of a factor of 3:** This corresponds to multiplying the entire function by 3 outside the exponent.

- Match:
`\( g(x) = 3(5)^x \)`

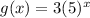
B. **Vertical compression of a factor of 1/3:** This corresponds to multiplying the entire function by
`\( (1)/(3) \)` outside the exponent.

- Match:
`\( g(x) = (1)/(3)(5)^x \)`

C. **Horizontal stretch of a factor of 3:** This corresponds to replacing
`\( x \) with \( (x)/(3) \)` inside the exponent.

- Match:
`\( g(x) = 5^{(1)/(3)x} \)`

D. **Horizontal compression of a factor of 1/3:** This corresponds to replacing x with 3x inside the exponent.

- Match:
`\( g(x) = 5^(3x) \)`

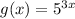
So, the correct matches are:

1. A -
`\( g(x) = 3(5)^x \)`

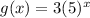
2. B -
`\( g(x) = (1)/(3)(5)^x \)`

3. C -
`\( g(x) = 5^{(1)/(3)x} \)`

4. D -
`\( g(x) = 5^(3x) \)`

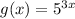
The probable question may be:

Consider this function.

f(x) = 5ˣ

How is function f transformed to create function g?

Match each transformation of function f with its description.

A. vertical stretch of a factor of 3

B. vertical compression of a factor of 1/3

C. horizontal stretch of a factor of 3

D. horizontal compression of a factor of 1/3

Options:

1. g(x) = 3(5)ˣ

2. g(x) = 1/3(5)ˣ

3. g(x) = 5^1/3x

4. g(x) = 5³ˣ