In the parallelogram OXYZ, with OX = a and OY = b, points P and R on OX and OY respectively are established. The simplified ratio ZP:ZR is (5a - 12b) : (8a + 3b).
In the parallelogram OXYZ, let's denote the point P on OX such that OP:PX = 1:2, and the point R on OY such that OR:RY = 1:3.
1. Express OP and PX in terms of a:
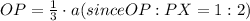
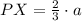
2. Express OR and RY in terms of b:
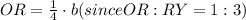
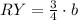
Now, we want to find the ratio ZP:ZR, where Z is the intersection of PR and QY.
ZP = OP - OZ
ZR = RY - OZ
3. Express OZ in terms of a and b:

Now, substitute these values into ZP and ZR:
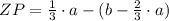
![\[ ZR = (3)/(4) \cdot b - (b - (2)/(3) \cdot a) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/hio3yol9bz8en8d3kmdsh51rwd12hnel6n.png)
4. Simplify the expressions:
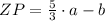
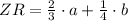
5. Find the ratio ZP:ZR:
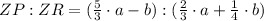
So, the simplified ratio ZP:ZR is (5a - 12b) : (8a + 3b).