Question

PLEASE HELP ASAP!!!

Amy has two accounts from which to choose to invest $3500. Account A offers 2.25% annual interest compounded quarterly. Account B offers continuous compound interest at the same interest rate. Amy plans to leave her investment untouched (no further deposits and no withdrawals) for 15 years.

1. Which account will yield the greater balance at the end of 15 years?

2. How much more money does Amy earn by choosing this more profitable account?​

Answer 1

1. Account B

2. $4.64

Explanation:

Amy wants to invest $3,500 for a period of 15 years, and has two accounts to choose from. To determine which account will yield the greater balance at the end of 15 years, we need to use an interest formula that is appropriate for the type of account.

Account A

Account A offers 2.25% annual interest compounded quarterly. Therefore, to calculate the amount in account A, we can use the compound interest formula:

`\boxed{\begin{array}{l}\underline{\textsf{Compound Interest Formula}}\\\\A=P\left(1+(r)/(n)\right)^(nt)\\\\\textsf{where:}\\\phantom{ww}\bullet\;\;\textsf{$A$ is the final amount.}\\\phantom{ww}\bullet\;\;\textsf{$P$ is the principal amount.}\\\phantom{ww}\bullet\;\;\textsf{$r$ is the interest rate (in decimal form).}\\\phantom{ww}\bullet\;\;\textsf{$n$ is the number of times interest is applied per year.}\\\phantom{ww}\bullet\;\;\textsf{$t$ is the time (in years).}\end{array}}`

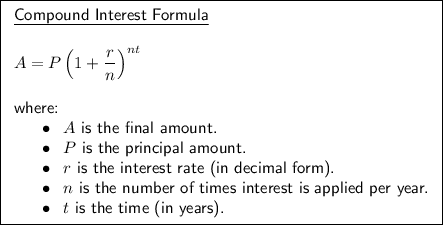
In thie case:

• P = $3,500
• r = 2.25% = 0.0225
• n = 4 (quarterly)
• t = 15 years

Substitute the values into the formula and solve for A:

`A=3500\left(1+(0.0225)/(4)\right)^(4 \cdot 15)`

`A=3500\left(1.005625\right)^(60)`

`A=3500\left(1.400114929666...\right)`

`A=4900.40\; \sf (2\;d.p.)`

Therefore, account A will yield a balance of $4,900.40 at the end of 15 years.

Account B

Account B offers continuous compound interest at 2.25%. Therefore, to calculate the amount in account B, we can use the continuous compounding interest formula:

`\boxed{\begin{array}{l}\underline{\textsf{Continuous Compounding Interest Formula}}\\\\A=Pe^(rt)\\\\\textsf{where:}\\\phantom{ww}\bullet\;\;\textsf{$A$ is the final amount.}\\\phantom{ww}\bullet\;\;\textsf{$P$ is the principal amount.}\\\phantom{ww}\bullet\;\;\textsf{$e$ is Euler's number.}\\\phantom{ww}\bullet\;\;\textsf{$r$ is the interest rate (in decimal form).}\\\phantom{ww}\bullet\;\;\textsf{$t$ is the time (in years).}\end{array}}`

In thie case:

• P = $3,500
• e = 2.718281828459...
• r = 2.25% = 0.0225
• t = 15 years

Substitute the values into the formula and solve for A:

`A=3500e^(0.0225 \cdot 15)`

`A=3500e^(0.3375)`

`A=3500(1.40143960839...)`

`A=4905.04\; \sf (2\;d.p.)`

Therefore, account B will yield a balance of $4,905.04 at the end of 15 years.

So, account B will yield the greater balance at the end of 15 years.

To calculate how much more money Amy will earn if she chooses account B, simply subtract the amount account A will yield from the amount account B will yield:

`\sf \$4905.04 - \$4900.40=\$4.64`

So, Amy will earn an additional $4.64 by choosing account B.

Answer 2

1) Account B

2) $127

Explanation:

Calculate the final balance for both accounts and then compare.

Account A:

P = $3500

r = rate of interest = 2.25% = 0.025

n = number of times interest is compounded in a year

= 4 times (as it is quarterly)

t = Number of years = 15 years

`\boxed{\bf A = P * \left(1 + (r)/(n)\right)^(nr)}`

`\sf = 3500 *\left(1 +(0.0225)/(4)\right)^(4*15)\\\\\\= 3500 * (1+ 0.005625)^(60)\\\\\\= 3500 * (1.005625)^(60)\\\\= \$ 4900.40`

= $4900

Account B:

P= $3500

Euler's constant = 2.9234

r = 0.0225

t = number of years = 15 years

`\boxed{\bf A_1 = P*(Euler's constant)^(rt)}`

`\sf = 3500 * (2.9234)^(0.0225*15)\\\\= 3500 * (2.9234)^(0.3375)\\\\= 5026.95\\\\=\$5027`

1) Account B yields the greater balance.

2) 5027 - 4900 = $ 127

Amy earns $127 more by choosing the profitable account.