Answer:
3 tiles will not fit together.
Explanation:
Measure of an Interior angle of a polygon =

Here, n = number of sides of the polygon
Therefore, measure of the interior angles of a regular hexagon,
A =
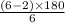
A = 120°
Similarly, interior angle of the regular pentagon,
B =
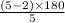
B = 108°
Now m∠A + m∠B + m∠C = 360°
m∠C = 360° - (120° + 108°)
= 132°
To fit the given three tiles perfectly, interior angle (∠D) of the third Octagonal tile should be 132°.
D =
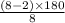
D = 135°
m∠C ≠ m∠D
Therefore, 3 tiles will not fit together.