Question :
The angle measure of a triangle are shown in the diagram.
What is the value of x?
Answer :
Explanation :
Given :
- ∠A = 50°
- ∠B = (2x)°
- ∠C = (3x - 10)°
To Find :
Solution :
As, In a triangle measure of all three angles is always 180°.


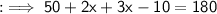
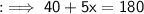

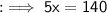
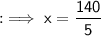

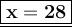
Hence, value of x is 28°.