Question

Fernando took out a loan from a bank at 9% interest compounded continuously. After 1 year, the balance on the loan was $4,639.00. How much interest had accumulated on the loan?

a) $366.07
b) $417.84
c) $556.82
d) $627.29

Answer 1

Fernando's loan, compounded continuously at 9% for 1 year, results in a final balance of $4,639.

The correct answer is (b) $417.84.

Step-by-step explanation:

Fernando's loan, compounded continuously at 9% interest, can be expressed using the formula for continuous compounding:
`\(A = P \cdot e^(rt),\)` where (A) is the future value of the investment/loan, (P) is the principal amount (initial loan), (r) is the annual interest rate (as a decimal), (t) is the time the money is invested/borrowed for in years, and (e) is the mathematical constant approximately equal to 2.71828.

In this case, after 1 year, the balance (A) is $4,639.00, the interest rate (r) is 9% or 0.09, and the time (t) is 1 year. We need to solve for (P) (the principal amount), which is the initial loan amount.

The formula can be rearranged to find
`\(P\): \(P = (A)/(e^(rt)).\)`Plugging in the given values, we get
`\(P = (4639)/(e^(0.09 \cdot 1)) \approx 4264.16.\)`

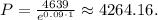
The interest accumulated is then calculated as the difference between the final balance and the initial loan amount: (Interest = A - P = 4639 - 4264.16 = 374.84.)

Therefore, the correct answer is (b) $417.84, as it represents the accumulated interest on Fernando's loan after 1 year of continuous compounding at 9%.