Question

State the Equation and the Domain and Range for the Absolute Value Graph given (Use lowercase T to make the absolute value. Do not use spaces in your equation) у Equation: f(x)= 1]X-2 +3

Answer 1

The equation for the absolute value graph is
`\( f(x) = |x - 2| + 3 \),` and the domain is all real numbers
`(\( x \in \mathbb{R} \)).` The range is
`\( f(x) \geq 3 \)` since the absolute value of any real number is non-negative, and adding 3 ensures that the range starts from 3.

Step-by-step explanation:

The given equation
`\( f(x) = |x - 2| + 3 \)` represents an absolute value function where the expression
`\( |x - 2| \)` ensures that the function takes the absolute value of ( x - 2 ). This absolute value function shifts the graph horizontally by 2 units to the right.

For the domain, since
`\( |x - 2| \)` is valid for all real numbers, the domain of
`\( f(x) \) is also all real numbers (\( x \in \mathbb{R} \)).`

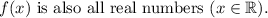
The range is determined by the absolute value part, which is always non-negative. Adding 3 to this ensures that the lowest value the function can take is 3. Therefore, the range is
`\( f(x) \geq 3 \).`

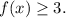
In summary, the equation
`\( f(x) = |x - 2| + 3 \)` describes an absolute value graph that is defined for all real numbers, and its range starts from 3 and goes upward.