Final Answer:
To find the value of ( x ) so that ( BC ) is parallel to ( DE ), we need to ensure that the slopes of the two lines are equal. Therefore, calculate the slope of line segment ( BC ) and line segment ( DE ) using their respective endpoints, then set them equal to each other and solve for ( x ).
Step-by-step explanation:
The slope ( m ) of a line passing through two points
 is given by the formula:
is given by the formula:
![\[ m = (y_2 - y_1)/(x_2 - x_1) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/vqy3fq0cgolowv3xchcub0teygxjk7luxm.png)
For line segment ( BC ), with points
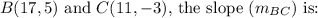
![\[ m_(BC) = (-3 - 5)/(11 - 17) = (-8)/(-6) = (4)/(3) \]](https://img.qammunity.org/2024/formulas/social-studies/high-school/nznvtrxepe3qp3g15ohzb4ob45ure64cvl.png)
For line segment ( DE ), with points ( D(-1, 2) ) and ( E(x, -6) ), the slope
 is calculated in terms of ( x ):
is calculated in terms of ( x ):
![\[ m_(DE) = ((-6 - 2))/((x - (-1))) = (-8)/((x + 1)) \]](https://img.qammunity.org/2024/formulas/social-studies/high-school/urztuox6v0n0i0avjz7t8gvcd1lufabo11.png)
Since ( BC ) is parallel to ( DE ), their slopes must be equal:
![\[ (4)/(3) = (-8)/((x + 1)) \]](https://img.qammunity.org/2024/formulas/social-studies/high-school/z6bj6v76uu4g40kypoqgm0de5zwty3qu4a.png)
Cross-multiply and solve for ( x ):
[ 4(x + 1) = -24 ]
[ 4x + 4 = -24 ]
[ 4x = -28 ]
[ x = -7 ]
Therefore, the value of ( x ) is -7 to make ( BC ) parallel to ( DE ).