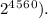Question

Evaluate the expression (28.3−5⋅60)− 2⁻²/2³​ raised to the power of 4⋅228, and provide your answer in simplified form.

Answer 1

To evaluate the expression, first simplify the expression within the parentheses. Then, evaluate the exponent. Finally, raise the previous result to the power of 4 * 228. The simplified form of the expression is 1 / (2^4560).

Step-by-step explanation:

To evaluate the expression, we must follow the order of operations: parentheses, exponents, multiplication and division (from left to right), and finally addition and subtraction (from left to right).

First, let's simplify the expression within the parentheses: 28.3 - 5 * 60 = 28.3 - 300 = -271.7.

Next, let's evaluate the exponent:
`2^-2/2^3`= 1/4 / 8 = 1/32.

Finally, let's raise the previous result to the power of 4 * 228: (1/32)^(912) = 1 /
`(32^9^1^2`) = 1 /
`((2^5)^9^1^2`) = 1 / (
`2^(5 * 912))` = 1 /
`(2^4^5^6^0)`.

So the simplified form of the expression is 1 / (
`2^4^5^6^0).`