Question

The exponential distribution has the probability function (y;)=1/ * exp(−y/) for >0 and y>0. Show the likelihood ratio test statistic for testing H0: =1.

Answer 1

The likelihood ratio test statistic for testing
`\(H_0: \lambda = \lambda_1\) is \(2 \lambda_1 \sum_(i=1)^(n) y_i\).`

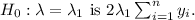
Step-by-step explanation:

In statistical hypothesis testing, the likelihood ratio test evaluates the likelihood of the null hypothesis
`\(H_0\)` against an alternative hypothesis by comparing their likelihood functions. For the exponential distribution, the likelihood function is \
`(L(\lambda) = (1)/(\lambda^n) e^{-(1)/(\lambda)\sum_(i=1)^(n) y_i}\), where \(\lambda\) is the parameter and \(y_i\)` are observed values. To test
`\(H_0: \lambda = \lambda_1\),`we construct the likelihood ratio test statistic.

The likelihood under the null hypothesis, denoted as
`\(L(\lambda_1)\), is \(L(\lambda_1) = (1)/(\lambda_1^n) e^{-(1)/(\lambda_1)\sum_(i=1)^(n) y_i}\).`The likelihood under the alternative hypothesis is \(L(\hat{\lambda}) =
`\frac{1}{\hat{\lambda}^n} e^{-\frac{1}{\hat{\lambda}}\sum_(i=1)^(n) y_i}\), where \(\hat{\lambda}\)` is the maximum likelihood estimate of
`\(\hat{\lambda}\)`

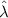
The likelihood ratio test statistic is the ratio of the likelihoods under the null and alternative hypotheses, i.e.,
`\( \Lambda = \frac{L(\lambda_1)}{L(\hat{\lambda})} \)`. Simplifying this ratio gives
`\( \Lambda = \frac{\lambda_1^n}{\hat{\lambda}^n} e^{-\frac{\hat{\lambda}-\lambda_1}{\hat{\lambda}\lambda_1}\sum_(i=1)^(n) y_i} \).` Taking the logarithm of this ratio yields
`\( -2\log{\Lambda} = 2 \lambda_1 \sum_(i=1)^(n) y_i \).`

This
`\( -2\log{\Lambda} \)` statistic follows a chi-squared distribution with 1 degree of freedom. It is used to decide whether to reject or fail to reject the null hypothesis based on the significance level and critical values.