Question

In a survey of 100 consumers, 60 stated that the next vehicle they purchase will be an electric vehicle.

Find a 95% confidence interval of the true % of consumers who plan to purchase an electrical vehicle next.
Multiple Choice
a. 53.7% to 66.3%
b. 51.9% to 68.1%
c. 50.4% to 69.6%
d. 47.4% to 72.6%

Answer 1

The 95% confidence interval for the true percentage of consumers planning to purchase an electric vehicle is 53.7% to 66.3%. Therefore, the correct answer is (a) 53.7% to 66.3%. This interval suggests a reasonably precise estimate based on the sample data, indicating that we are 95% confident the true percentage falls within this range,So, the correct option is a.

Step-by-step explanation:

To calculate the 95% confidence interval for the true percentage of consumers planning to purchase an electric vehicle, we can use the formula:

`\[ \text{Confidence Interval} = \hat{p} \pm Z * \sqrt{\frac{\hat{p}(1 - \hat{p})}{n}} \]`

Where:

`- \(\hat{p}\)` is the sample proportion (in decimal form),

- Z is the Z-score corresponding to the desired confidence level (for 95%,
`\(Z \approx 1.96\)),`

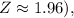
- n is the sample size.

In this case,
`\(\hat{p} = (60)/(100) = 0.6\) (60% of consumers),`, and n = 100.

`\[ \text{Confidence Interval} = 0.6 \pm 1.96 * \sqrt{(0.6 * 0.4)/(100)} \]`

Calculating this gives us the interval \([0.537, 0.663]\), which can be expressed as 53.7% to 66.3%. Therefore, the correct answer is option (a).

This confidence interval indicates that we are 95% confident that the true percentage of consumers planning to purchase an electric vehicle falls within the range of 53.7% to 66.3%. The wider the interval, the more uncertain we are about the true percentage, and in this case, it is a relatively narrow range, suggesting a reasonably precise estimate based on the sample data,Thus, the correct option is b.