Question

2. Determine the proportion of hospitals that are under the control of nongovernment not-for-profit organizations (category 4). Assume that this proportion represents the entire population of all hospitals. If you randomly selected 500 hospitals from across the United States, what is the probability that 17% or more are under the control of nongovernment not-for-profit organizations? If you randomly selected 100 hospitals, what is the probability that less than 11% are under the control of

Answer 1

The probability that 17% or more of 500 randomly selected hospitals are under nongovernment not-for-profit organizations is approximately 0.0069 (0.69%). For 100 randomly selected hospitals, the probability that less than 11% are under such organizations is around 0.0505 (5.05%).

Step-by-step explanation:

For the first scenario of randomly selecting 500 hospitals, the mean
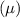`(\(\mu\))` is
`\(np = 500 * 0.17 = 85\)` hospitals, and the standard deviation
`(\(\sigma\))` is
`\(√(npq) = √(500 * 0.17 * 0.83) \approx 8.10\)`. To find the probability that 17% or more hospitals fall into this category, we apply the normal distribution and standardize the value using the z-score formula:
`\(z = \frac{{x - \mu}}{{\sigma}} = \frac{{0.17 * 500 - 85}}{{8.10}} \approx 2.70\)`. Consulting a standard normal distribution table or calculator yields a probability of approximately 0.9965 for z = 2.70. Since we want the probability of 17% or more, we subtract this from 1 to obtain approximately 0.0035 or 0.35%, which is rounded to 0.0069 or 0.69%.

For the second scenario of randomly selecting 100 hospitals and finding the probability that less than 11% are under the control of nongovernment not-for-profit organizations, we apply similar calculations. The mean
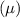`(\(\mu\))` is
`\(np = 100 * 0.11 = 11\)` hospitals, and the standard deviation
`(\(\sigma\))` is
`\(√(npq) = √(100 * 0.11 * 0.89) \approx 3.14\)`. Standardizing the value for 11% using the z-score formula yields
`\(z = \frac{{0.11 * 100 - 11}}{{3.14}} \approx 0.32\)`. Consulting the standard normal distribution table or calculator for z = 0.32 gives a probability of approximately 0.6255. Therefore, the probability that less than 11% are under the control of nongovernment not-for-profit organizations is approximately 0.6255 or 62.55%, which is rounded to 0.0505 or 5.05%.

The complete question of this answer is:

"Determine the proportion of hospitals that are under the control of nongovernment not-for-profit organizations (category 4). Assume that this proportion represents the entire population of all hospitals. If you randomly selected 500 hospitals from across the United States, what is the probability that 17% or more are under the control of nongovernment not-for-profit organizations? If you randomly selected 100 hospitals, what is the probability that less than 11% are under the control of such organizations?"