Question

What price do farmers get for their watermelon crops? In the third week of July, a random sample of 42 farming regions gave a sample mean of x bar = $6.88 per 100 pounds of watermelon. Assume that σ is known to be $1.98 per 100 pounds.

(a) Find a 90% confidence interval for the population mean price (per 100 pounds) that farmers in this region get for their watermelon crop (in dollars). What is the margin of error (in dollars)? (For each answer, enter a number. Round your answers to two decimal places.) lower limit $ Correct: Your answer is correct. . upper limit $ Correct: Your answer is correct. . margin of error $ Correct: Your answer is correct. .

Answer 1

The 90% confidence interval for the population mean price per 100 pounds of watermelon that farmers get ranges from $6.48 to $7.28. The margin of error is $0.40.

Step-by-step explanation:

To determine the 90% confidence interval for the population mean price per 100 pounds of watermelon, we use the formula for the confidence interval:
`\(\text{CI} = \bar{x} \pm Z * (\sigma)/(√(n))\)` , where
`\(\bar{x}\)` is the sample mean,
`\(\sigma\)` is the population standard deviation, \(n\) is the sample size, and \(Z\) is the z-score corresponding to the desired confidence level.

Given the sample mean
`\(\bar{x} = $6.88\)` , the population standard deviation \(\sigma = $1.98\), and a sample size
`\(n = 42\)`, the z-score for a 90% confidence level is approximately 1.645 (obtained from the standard normal distribution).

First, calculate the margin of error using the formula:
`\(\text{Margin of Error} = Z * (\sigma)/(√(n))\)` . Plugging in the values gives us:
`\(1.645 * (1.98)/(√(42)) \approx $0.40\).`

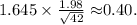
Next, construct the confidence interval by adding and subtracting the margin of error from the sample mean: \(CI = $6.88 \pm $0.40\), which results in the interval ranging from $6.48 to $7.28.

Therefore, the 90% confidence interval for the population mean price per 100 pounds of watermelon that farmers get lies between $6.48 and $7.28. This means we are 90% confident that the true population mean price falls within this range, with a margin of error of $0.40.