Question

A survey is planned to determine the mean annual family medical expenses of employees of a large company. The management of the company wishes to be 95% confident that the sample mean is correct to within ±$50 of the population mean annual family medical expenses. A previous study indicates that the standard deviation is approximately $400.

a. How large sample is necessary?
b. If management wants to be correct to within ±$25 how many employees need to be selected?

Answer 1

a. To be 95% confident that the sample mean is correct to within ±$50, a sample size of approximately 246 is necessary.

b. If management wants to be correct to within ±$25, the required sample size would be approximately 983.

Step-by-step explanation:

a. The formula for calculating the required sample size
`(\(n\))` to estimate the population mean with a specified margin of error is given by:

`\[ n = \left((Z \cdot \sigma)/(E)\right)^2 \]`

where:

-
`\(Z\)` is the Z-score corresponding to the desired level of confidence,

-
`\(\sigma\)` is the standard deviation,

-
`\(E\)`is the desired margin of error.

For a 95% confidence level,
`\(Z\)` is approximately 1.96. Substituting the values:

`\[ n = \left((1.96 \cdot 400)/(50)\right)^2 \]`

Solving for \(n\), we get \(n \approx 245.96\). Since the sample size must be a whole number, round up to the nearest whole number, which gives
`\(n \approx 246\).`

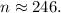
b. For a margin of error of ±$25, the formula becomes:

`\[ n = \left((1.96 \cdot 400)/(25)\right)^2 \]`

Solving for
`\(n\)`, we get
`\(n \approx 982.4\).` Rounding up to the nearest whole number, the required sample size is approximately 983.

In summary:

a. A sample size of 246 is necessary for a ±$50 margin of error.

b. A sample size of 983 is necessary for a ±$25 margin of error.