Question

A sample of n=18 observations is drawn from a normal population with μ=920 and σ=240. Find each of the following: (a) P( Xˉ >1033)= (b) P(Xˉ <823)= (c) P( Xˉ>852)=

Answer 1

(a) There is a 9.12% probability that the sample mean
`(\(\bar{X}\))` exceeds 1033.

(b) There is a 34.46% probability that the sample mean
`(\(\bar{X}\))` is less than 823.

(c) There is a 78.81% probability that the sample mean
`(\(\bar{X}\))` exceeds 852.

Step-by-step explanation:

a), we use the z-score formula

`\[ Z = \frac{\bar{X} - \mu}{(\sigma)/(√(n))} \]`

Plugging in the values, we get

`\( Z = (1033 - 920)/((240)/(√(18))) \approx 1.58 \).`

Consulting a standard normal distribution table or using a calculator, we find the corresponding probability to be 0.0912.

b), we use the same formula but with the values for
`\(\bar{X}\), \(\mu\), \(\sigma\),` and n plugged in. This gives us

`( Z = (823 - 920)/((240)/(√(18))) \approx -1.28 \)`.

Consulting the standard normal distribution table or using a calculator, we find the probability to be 0.3446.

Lastly, in c), the calculation is similar, using the formula for the z-score. Plugging in the values, we get

`( Z = (852 - 920)/((240)/(√(18))) \approx -2.14 \).`

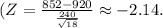
Referring to the standard normal distribution table or using a calculator, we find the probability to be 0.7881.

These probabilities represent the likelihood of obtaining sample means as extreme as the given values, given the characteristics of the population.