Question

3. Suppose an investment of P100,000 was made, and it matured to P104,070.70 after eight (8)

compounding periods at 2% interest. How many compounding periods were there in a year?
Rubric for scoring:


P.s: I only need the answer for number 3 and I need a serious one asap

Answer 1

Problem 3

Answer: 4 compounding periods per year

In other words, the money is being compounded quarterly

===========================================================

Step-by-step explanation:

The compound interest formula is

`A = P*(1+(r)/(n))^(n*t)\\\\`

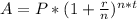
The variables are

• A = final amount after t years
• P = initial deposit, aka principal
• r = interest rate in decimal form
• n = number of compounding periods per year
• t = number of years

In this case, we have

• A = 104,070.70
• P = 100,000
• r = 0.02
• n = unknown (what we want to solve for)
• t = also unknown, but we do know that n*t = 8 because this represents the number of total compounding periods.

So,

`A = P*(1+(r)/(n))^(n*t)\\\\104,070.70 = 100,000*(1+(0.02)/(n))^(8)\\\\(1+(0.02)/(n))^(8) = (104,070.70)/(100,000)\\\\(1+(0.02)/(n))^(8) = 1.040707\\\\1+(0.02)/(n) = (1.040707)^(1/8)\\\\1+(0.02)/(n) \approx 1.0049999946977\\\\n+0.02 \approx 1.0049999946977n\\\\0.02 \approx 1.0049999946977n-n\\\\0.02 \approx 0.0049999946977n\\\\n \approx (0.02)/(0.0049999946977)\\\\n \approx 4.0000042418445\\\\n \approx 4`

So it appears that we're compounding the money n = 4 times a year (aka quarterly) and doing so for 8/4 = 2 years.

Note how,

`A = P*(1+(r)/(n))^(n*t)\\\\A = 100,000*(1+(0.02)/(4))^(4*2)\\\\A = 100,000*(1.005)^(8)\\\\A \approx 104,070.704392543\\\\A \approx 104,070.70\\\\`

which helps confirm the answer.