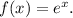Final Answer:
The function
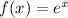 satisfies the Mean Value Theorem hypotheses on
satisfies the Mean Value Theorem hypotheses on
![\([0, \ln 4]\)](https://img.qammunity.org/2024/formulas/mathematics/high-school/vflc0rtz59twnhacc9e6kwlvxw42zdmzkj.png) , and
, and
 can be any real number in this interval. This is because the function is continuous and differentiable everywhere, meeting the conditions of the theorem. C. The function satisfies the hypotheses, and c can be any real number in the interval [0, ln 4].
can be any real number in this interval. This is because the function is continuous and differentiable everywhere, meeting the conditions of the theorem. C. The function satisfies the hypotheses, and c can be any real number in the interval [0, ln 4].
Step-by-step explanation:
The Mean Value Theorem (MVT) states that if a function
 is continuous on the closed interval
is continuous on the closed interval
![\([a, b]\)](https://img.qammunity.org/2024/formulas/mathematics/high-school/s202c8hurt2j2kwp3oixof8ryjk64ug9lb.png) and differentiable on the open interval
and differentiable on the open interval
 , then there exists at least one number
, then there exists at least one number
 in the interval
in the interval
 such that
such that
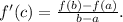
In our case, the function
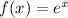 is continuous and differentiable for all real numbers. Thus, it satisfies the hypotheses of the MVT. The interval
is continuous and differentiable for all real numbers. Thus, it satisfies the hypotheses of the MVT. The interval
![\([0, \ln 4]\)](https://img.qammunity.org/2024/formulas/mathematics/high-school/vflc0rtz59twnhacc9e6kwlvxw42zdmzkj.png) is closed and
is closed and
 is open, so the conditions are met.
is open, so the conditions are met.
Now, to find
 , we calculate
, we calculate
 :
:
![\[ f'(x) = (d)/(dx)(e^x) = e^x \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/m5od2i3yoj8qjfxt9or4kinc1itqhw4xjr.png)
Apply the MVT formula:
![\[ e^c = (e^(\ln 4) - e^0)/(\ln 4 - 0) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/m6dai45atx0g9c34dzpdy362yrur3khvic.png)
Simplify:
![\[ e^c = (4 - 1)/(\ln 4) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/ifhyo43ysxlb9788zvuxegajpy7d8ijsf8.png)
Thus,
 can be any real number in the interval
can be any real number in the interval
![\([0, \ln 4]\)](https://img.qammunity.org/2024/formulas/mathematics/high-school/vflc0rtz59twnhacc9e6kwlvxw42zdmzkj.png) , and the correct option is C. The conclusion is that there exists at least one
, and the correct option is C. The conclusion is that there exists at least one
 in
in
![\([0, \ln 4]\)](https://img.qammunity.org/2024/formulas/mathematics/high-school/vflc0rtz59twnhacc9e6kwlvxw42zdmzkj.png) satisfying the MVT for
satisfying the MVT for