Question

The drawing shows a person who, starting from rest at the top of a cliff, swings down at the end of a rope, releases it, and falls into the water below. There are two paths by which the person can enter the water. Suppose he enters the water at a speed of 11.6 m/s via path 1. How fast is he moving on path 2 when he releases the rope at a height of 3.24 m above the water? Ignore the effects of air resistance.

Answer 1

The speed of the person at path 2, given that he releases the rope at a height of 3.24 m above the water, is 7.94 m/s

How to determine the speed at path 2?

This type of motion is described as motion under gravity.

Thus, we shall start our calculation by calculating the time taken for the person to fall from the height of 3.24 m into the water. This is shown below:

• Height above the water (h) = 3.24 m
• Acceleration due to gravity (g) = 9.8 m/s²
• Time taken (t) = ?

`t = \sqrt{(2h)/(g)} \\\\t = \sqrt{(2\ *\ 3.24)/(9.8)}\\\\t = 0.81\ s`

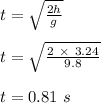
With the time calculated above, we can calculate the speed of the person at path 2. Details below

• Initial velocity of object (u) = 0 m/s (Since he is falling)
• Time taken (t) = 0.81 s
• Acceleration due to gravity (g) = 9.8 m/s²
• Velocity of person at path 2 (v) =?

v = u+ gt

v = 0 + (9.8 × 0.81)

v = 0 + 7.94

= 7.94 m/s

Missing part:

See attached photo