Final answer:
After evaluating each ordered pair, (5, –2) and (3, 1) satisfy both inequalities and are part of the solution set, but (–4, 2) does not and is excluded.
Step-by-step explanation:
We need to determine which ordered pairs satisfy both inequalities
 This means we are looking for points that are above the line with a negative slope (a) and below the line with a positive slope (b).
This means we are looking for points that are above the line with a negative slope (a) and below the line with a positive slope (b).
To check each point, substitute the x and y values into both inequalities:
- (5, –2):
 becomes
becomes
 which simplifies to
which simplifies to
 ), which is true. y < \(\frac{1}{2}\)x + 1 becomes –2 < \(\frac{1}{2}\)(5) + 1, which simplifies to –2 < 3.5, which is also true. So, (5, –2) is a solution.
), which is true. y < \(\frac{1}{2}\)x + 1 becomes –2 < \(\frac{1}{2}\)(5) + 1, which simplifies to –2 < 3.5, which is also true. So, (5, –2) is a solution.
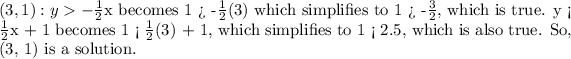
 becomes
becomes
 which simplifies to 2 > 2, which is not true. So, (–4, 2) is not a solution.
which simplifies to 2 > 2, which is not true. So, (–4, 2) is not a solution.
Therefore, the ordered pair (5, –2) and (3, 1) are in the solution set of the system, but (–4, 2) is not.