Question

Given the following exponential function, identify whether the change represents growth or decay, and determine the percentage rate of increase or decrease. y= 8600(0.11)x

Answer 1

The change represents a decay, and the percentage rate of decrease is 89%.

In Mathematics and Statistics, a population that increases at a specific period of time represent an exponential growth.

This ultimately implies that, a mathematical model for any population that increases by r percent per unit of time is an exponential function of this form:

`P(t) = I(1 + r)^t`

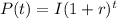
Where:

• P(t ) represents the final population.
• t represents the time or number of years or days.
• I represents the initial population.
• r represents the growth rate.

Based on the exponential functions provided above, we can reasonably infer and logically deduce that the required exponential growth rate function is given by;

`y = 8600(0.11)^x`

1 + r = 0.11

r = 0.11 - 1

r = -0.89 × 100

r = -89%