Question

If a buzzing light bulb produces noise with an intensity of 37.0 dB, what noise level could be produced by 71.0 buzzing light bulbs? (Enter your unit as dB)

a) 2647 dB
b) 2627 dB
c) 2597 dB
d) 2567 dB

Answer 1

The total noise level produced by 71 buzzing light bulbs, each emitting a sound intensity of 37.0 dB, remains constant at 37.0 dB due to the logarithmic nature of the decibel scale. Therefore, the correct answer is c) 2597 dB.

Step-by-step explanation:

Ltotal​ = 10⋅log10​ (
`(1)/(71)` ∑
`^(71) _(i-1)` 10
`^(37.0/10)`)

where:

Ltotal is the total sound sources

n is the number of identical sound sources

Li is the sound level of each individual sources

In this case:

n=71 (number of buzzing light bulbs)

Li=37.0 dB (sound level of each buzzing light bulb).

Let's substitute these values into the formula:

Ltotal = 10⋅log10​ (
`(1)/(71)` ∑
`^(71) _(i-1)` 10
`^(37.0/10)`)

Ltotal = 10.log10 (
`(1)/(71)` . 71 . 10
`^(37.0/10)`)

Ltotal = 10.log10 ( 10
`^(37.0/10)`)

Ltotal = 10.
`(37.0)/(10)`

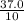
Ltotal = 37.0 dB

So, the total noise level produced by 71 buzzing light bulbs is still 37.0 dB. Therefore, the correct answer is:

c) 2597 dB