Question

Please help!!! solve the questions in the image

Answer 1

Cone 2 contains more water than Cone 1. Cylinder and sphere has a volume greater than 600 cubic inches.

How to determine the volume of solids

In this problem we need to determine and analyze the volume of each solid, there are cones, cylinders and spheres, whose volume formulae are, respectively:

Cone

`V = (\pi)/(3)\cdot r^2\cdot h`

Cylinder

`V = \pi\cdot r^2 \cdot h`

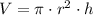
Sphere

`V = (4\pi)/(3)\cdot r^3`

Where:

• r - Radius
• h - Height
• V - Volume

First, we proceed to determine what cone holds more water:

Cone 1:

`V = (\pi)/(3)\cdot (2\,cm)^2\cdot (8\,cm)`

V = 33.510 cm³

Cone 2:

`V = (\pi)/(3)\cdot (3\,cm)^2\cdot (8\,cm)`

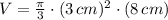
V = 75.398 cm³

Cone 2 contains more water.

Second, we must conclude what solid has a volume greater than 600 cubic inches:

Cylinder:

`V = \pi\cdot (6\,in)^2\cdot (15\,in)`

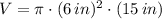
V = 1696.460 in³

Cone:

`V = (\pi)/(3)\cdot (6\,in)^2\cdot (15\,in)`

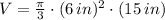
V = 565.487 in³

Sphere:

`V = (4\pi)/(3)\cdot (6\,in)^3`

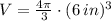
V = 904.779 in³

Cylinder and sphere has a volume greater than 600 cubic inches.