Question

The expression (x^13)(x^6) is equivalent to x^p. What is the value of p?

Answer 1

p=19

Explanation:

since the instructions say that (
`x^(13)`)(
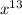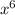`x^(6)`) is equivalent to
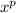`x^(p)`, we can make the equation:

(
`x^(13)`)(
`x^(6)`)=
`x^(p)`

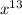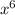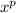
now, apply the laws of exponents;
`a^(m) * a^(n) =a^(m+n)`

in that case, since we're multiplying x to the 13th power by x to the 6th, the result is
`x^(13+6)`, which is
`x^(19)`

the equation now:

`x^(19)`=
`x^(p)`

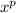
since the exponents are at the same base, we can solve the equation

19=p

so p is 19

hope this helps :)