Question

30 POINTS

Identify the following for the exponential function y = 5(1/4)x.

a. Growth or decay?

b. Initial value

c. Growth/decay factor

d. Growth/decay rate (as a percentage)

Answer 1

A)

Decay

B)

Initial value is 5.

C)

The decay factor is 0.75.

D)

75%

Explanation:

An exponential function is given by:

`f(x)=a(r)^x`

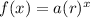
Where a is the initial value and r is the rate.

We have the function:

`y=5(1/4)^x`

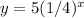
So, a = 5 and r = 1/4.

A)

Remember the guidelines:

• It is exponential growth if r > 1.
• And it is exponential decay if r < 1. (And r must be positive.)

Our rate is 1/4 or 0.25.

It is less than 1, so our function is exponential decay.

B)

The initial value is the value in front of our rate.

So, our initial value in this case is 5.

C)

The decay factor will be 1 - r, where r is our rate.

Since our rate is 0.25, our decay factor is 1 - 0.25 = 0.75.

So, we are decaying by a factor of 0.75.

D)

To convert a decimal to a percent, we move the decimal dot two places to the rght and add a percent sign.

So, 0.75 will be 75.0 % or smply 75%.

It is a 75% decay.