Final answer:
To determine the polynomial with the given roots, we solve the equation using the quadratic formula and compare the options.
Step-by-step explanation:
The given options are:
A)

B)
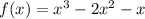
C)
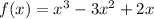
D)
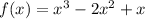
To determine which polynomial has the given roots, we need to solve the equation
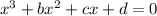 using the quadratic formula. The roots of the polynomial are the values of x that make the equation equal to zero. Comparing the given options with the quadratic formula, we find that option C)
using the quadratic formula. The roots of the polynomial are the values of x that make the equation equal to zero. Comparing the given options with the quadratic formula, we find that option C)
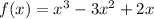 has the same roots as the given equation.
has the same roots as the given equation.