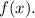Final Answer:
The expression that accurately represents
 for the given function
for the given function
 is option (c)
is option (c)
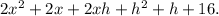
Explanation:
To determine
 we substitute
we substitute
 , replacing each
, replacing each
 with
with
 The expanded and simplified result is
The expanded and simplified result is
 . The correct option (c) precisely represents this expression, encompassing the correct terms and coefficients.
. The correct option (c) precisely represents this expression, encompassing the correct terms and coefficients.
Understanding the process of substituting variables and simplifying expressions is fundamental in evaluating functions with altered inputs.
In this scenario, option (c) accurately denotes the function
 for the provided quadratic function
for the provided quadratic function